Theory of electron and vibrational excitation
UV and VIS spectroscopy is based on the excitation of electrons in the outer electron shell (orbital) of an atom or molecule. The energy input of the excitation radiation transfers electrons from the highest occupied orbital - the ground state - to the lowest unoccupied orbital - the excited state. The energy required for this transition is obtained by absorption from the excitation radiation. Theoretically, the absorption of organic molecules can be well described by the so-called HOMO-LUMO concept (HOMO, highest occupied molecular orbital; LUMO, lowest unoccupied molecular orbital), while the absorption of elements (especially for the frequently colored compounds of groups 3-12 in the periodic table) is well described by the ligand field theory. Further absorption in the UV-VIS occurs in compounds due to free electron pairs or multiple bonds, among other things. In general, the more extended an electron system is, the less energy is required for electron excitation and the stronger the absorption is shifted to longer wavelengths (i.e. into visible light). These 'color-giving units' of the molecules are traditionally referred to as 'chromophores'. The electron transitions also follow certain selection rules, so that not all theoretically possible electronic transitions can actually be observed in every case (there are symmetry-prohibited transitions as well as spin-prohibited transitions).
Optical fluorescence spectroscopy is also based on the observation of electronic transitions, but during relaxation (transition of electrons from the excited state to the ground state), after absorption. Compared to directly observable absorption, the quantum yield of fluorescence is low. This is due to the fact that some of the electrons are excited to vibrate during absorption in addition to being excited to a higher orbital (vibronic excitation). However, this process only takes place for a small proportion of the excited electrons. As a result, these excited vibrations relax without radiation and the remaining amount of energy is emitted as fluorescent radiation at longer wavelengths when they return to the ground state.5 However, as the intensity of the fluorescence is directly proportional to the excitation radiation, the most intense radiation possible is used for excitation.
In IR spectroscopy, absorption of the sample is also observed based on the excitation radiation. However, the energy of the radiation excites vibrations between the atoms in the molecule or a solid lattice. From a theoretical point of view, the vibrational frequencies of the molecules can be described approximately using the spring force model as a harmonic oscillator. If the considerations are continued on the basis of wave mechanics and only the accessible, discrete energy levels are taken into account, the equation is obtained for diatomic molecules:
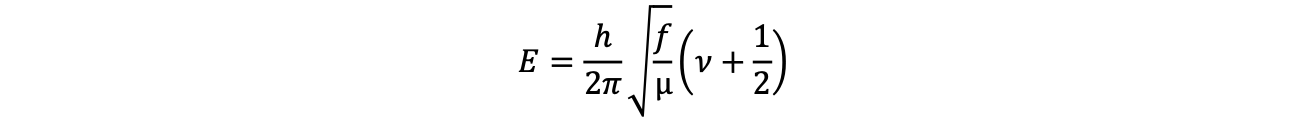
(E energy, h Planck's quantum of action, f force constant, μ reduced mass, ν oscillation quantum number)
In reality, the molecules do not behave completely harmonically (if the oscillation amplitudes are too large, the molecules would dissociate or break up the lattice), so that overtone oscillations do not occur entirely at twice the energy in the spectrum, contrary to the above equation. In multi-atomic molecules, the vibrations of the individual atoms are coupled and the theoretical description of the oscillations becomes much more complicated. However, the number of oscillations in a molecule can be determined very precisely using the following equation:6
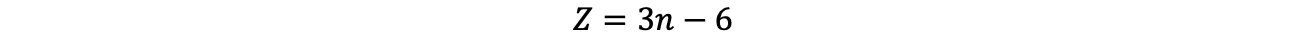
(Z number of vibrations, n number of atoms in the molecule; for linear molecules: Z = 3n - 5)
The types of vibrations are described using Greek letters and are fundamentally divided into valence vibrations (also known as stretching vibrations, νs symmetrical, νas antisymmetrical) and deformation vibrations (δ and γ bending vibrations, τ twisting-S., ρ rocking-S., ω wagging-S.). In IR spectroscopy, only those vibrations that lead to a change in the dipole moment of a molecule can be observed. Accordingly, the vibrations are divided into IR-active and IR-inactive. For IR spectroscopy, too, there are clearly more detailed considerations on the symmetry of the molecules or lattices as well as selection rules; here too, reference should be made to the literature already mentioned.
Complementary to IR spectroscopy is RAMAN spectroscopy, i.e. IR-inactive vibrations can be observed in RAMAN. In contrast to IR spectroscopy, those vibrations that lead to a change in the polarizability of the molecule are RAMAN-allowed. In this technique, also known as RAMAN scattering, a molecule is excited by monochromatic light in the visible or near-infrared spectral range. The energy introduced by the exciting radiation is usually re-emitted (by electron oscillations) while maintaining the frequency; this elastic scattering is called Rayleigh scattering. If the electron oscillations induced by the excitation lead to a change in the polarizability of the entire molecule, a small part of the energy introduced is used to excite the molecular oscillation and the inelastically scattered (Stokes scattering), frequency-reduced radiation is re-emitted. The scattering is diffuse in all spatial directions. The highest RAMAN intensities are observed in vibrations in which the entire molecule is contracted or stretched. An illustrative example is the symmetrical stretching vibration of CO2.
References:
5 For a complete description of the relaxation processes of fluorescence spectroscopy, please refer to further literature. I. e. M. Otto, „Analytische Chemie“, Wiley-VCH, 4. Auflage 2011, ISBN 9783527328819
6 A molecule with n atoms has 3n degrees of freedom of movement corresponding to the three spatial directions. Of these, 3 are allotted to translational motion and 3 to rotational motion (for linear molecules, rotation is identical for two spatial directions and only 2 degrees of freedom are deducted), in each case along the spatial axes. The remaining degrees of freedom are accounted for by the vibrational oscillation.
